Augmented Matrix Example Math

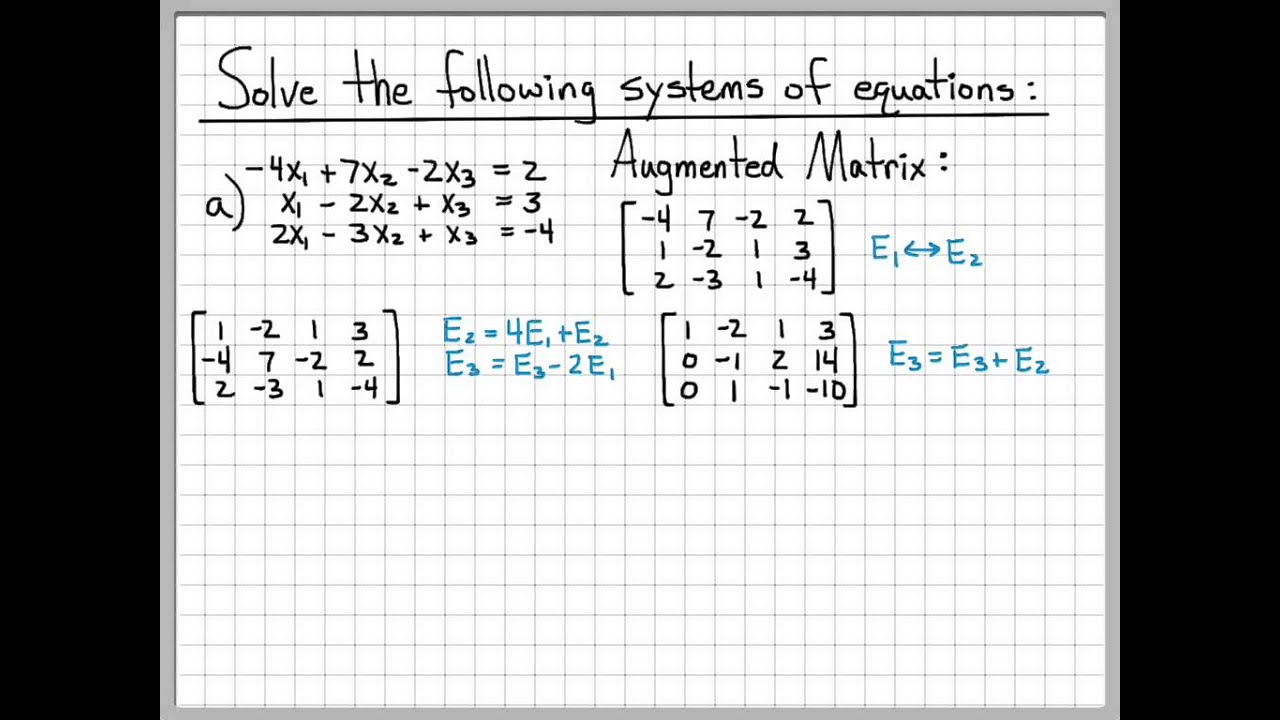
Row operations when a system of equations is in an augmented matrix we can perform calculations on the rows to achieve an answer.

Augmented matrix example math. We use a vertical line to separate the coefficient entries from the constants essentially replacing the equal signs. Perform the row operation on row in order to convert some elements in the row to. Let s take a look at an example. Due to the nature of the mathematics on this site it is best views in landscape mode.

To express a system in matrix form we extract the coefficients of the variables and the constants and these become the entries of the matrix. Systems of linear equations. The example above is a 2 variable matrix below is a three variable matrix. If your device is not in landscape mode many of the equations will run off the side of your device should be able to scroll to see them and some of the menu items will be cut off due to the narrow screen width.

It is created by adding an additional column for the constants on the right of the equal signs. A matrix can serve as a device for representing and solving a system of equations. Solve using an augmented matrix write the system of equations in matrix form. A matrix form of a linear system of equations obtained from the coefficient matrix as shown below.

In linear algebra an augmented matrix is a matrix obtained by appending the columns of two given matrices usually for the purpose of performing the same elementary row operations on each of the given matrices. Using row operations on an augmented matrix to solve a system of equations is called the gauss jordan method. In your example we only needed to perform a few row operations to get the system into a form which could be easily dealt with by observing that y 0. If you look closely you can see there is nothing here new except the z variable with its own column in the matrix.